Event segment
A segment or trajectory is a relation between an element of an arbitrary set 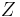 and a time of time base
and a time of time base 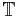 [Zeigler76] and [ZPK00]. As timed sequences of events, event segments are a special class of the general segment. Event segments are used to define Timed Event Systems such as DEVS, timed automata, and timed petri nets.
[Zeigler76] and [ZPK00]. As timed sequences of events, event segments are a special class of the general segment. Event segments are used to define Timed Event Systems such as DEVS, timed automata, and timed petri nets.
Contents |
Event segments
Event and null event
An event is a label that abstracts a change. Given an event set 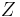 , the null event denoted by
, the null event denoted by 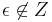 stands for nothing change.
stands for nothing change.
Time base
The time base of the concerning systems is denoted by 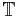 , and defined
, and defined
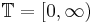
as the set of non-negative real numbers.
Timed event
A timed event  over an event set
over an event set 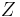 and the time base
and the time base 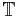 denotes that an event
denotes that an event 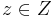 occurs at time
occurs at time 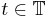 .
.
Null event segment
The null event segment over time interval ![[t_l, t_u] \subset \mathbb{T}](/2012-wikipedia_en_all_nopic_01_2012/I/583527256dedc28162634173789ce135.png) is denoted by
is denoted by ![\epsilon_{[t_l, t_u]}](/2012-wikipedia_en_all_nopic_01_2012/I/2a38e53fc1c66cf8992ed207dc41180e.png) which means that there is no event over
which means that there is no event over ![[t_l, t_u]](/2012-wikipedia_en_all_nopic_01_2012/I/38d72adb0dfab9d43f3efa610f1b58fc.png) .
.
Unit event segment
A unit event segment is either a null event segment or a timed event.
Concatenation
Given an event set 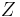 , concatenation of two unit event segments
, concatenation of two unit event segments 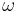 over
over ![[t_1, t_2]](/2012-wikipedia_en_all_nopic_01_2012/I/345dab1f62c55d4cbd787273bd257448.png) and
and 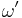 over
over ![[t_3,
t_4]](/2012-wikipedia_en_all_nopic_01_2012/I/0a04dada4e1aa7b4c333db8b8694318f.png) is denoted by
is denoted by 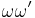 whose time interval is
whose time interval is ![[t_1,
t_4]](/2012-wikipedia_en_all_nopic_01_2012/I/ff8e4a62098de23f2e1f3ea49cce4cb2.png) , and implies
, and implies 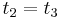 .
.
Multi-event segment
A multi-event segment  over an event set
over an event set 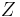 and a time interval
and a time interval ![[t_l, t_u] \subset \mathbb{T}](/2012-wikipedia_en_all_nopic_01_2012/I/583527256dedc28162634173789ce135.png) is concatenation of unit event segments
is concatenation of unit event segments ![\epsilon_{[t_l,t_1]},(z_1,t_1), \epsilon_{[t_1,t_2]},(z_2,t_2),\ldots, (z_n,t_n),](/2012-wikipedia_en_all_nopic_01_2012/I/ac150ffa9e3c64ff27c244572c8feea3.png) and
and ![\epsilon_{[t_n,t_u]}](/2012-wikipedia_en_all_nopic_01_2012/I/c7e5abaa8c1cf985e94a7b4e5ff3e132.png) where
where  .
.
Timed language
The universal timed language over an event set 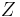 and a time interval
and a time interval ![[t_l, t_u] \subset \mathbb{T}](/2012-wikipedia_en_all_nopic_01_2012/I/583527256dedc28162634173789ce135.png) , is denoted by
, is denoted by ![\Omega_{Z,[t_l, t_u]}](/2012-wikipedia_en_all_nopic_01_2012/I/5f3d753b7e2e45eab8f07286ba0b9b2d.png) , and is defined as the set of all possible event segments. Formally,
, and is defined as the set of all possible event segments. Formally,
![\Omega_{Z,[t_l,t_u]}=\{(z,t)^*| z \in Z \cup \{\epsilon\}, t \in [t_l, t_u] \}](/2012-wikipedia_en_all_nopic_01_2012/I/4e1ba453fb72cc83ab2424071f2c012c.png)
where  denotes a none or multiple concatenation(s) of timed events. Notice that the number of events in an event segment
denotes a none or multiple concatenation(s) of timed events. Notice that the number of events in an event segment ![\omega \in
\Omega_{Z,[t_l, t_u]}](/2012-wikipedia_en_all_nopic_01_2012/I/eb8db71e91094eeb0c082d3bd74429cf.png) can be one of zero, finite or infinite. Infinitely many events in an event segment
can be one of zero, finite or infinite. Infinitely many events in an event segment ![\omega \in \Omega_{Z,[t_l,
t_u]}](/2012-wikipedia_en_all_nopic_01_2012/I/eb8db71e91094eeb0c082d3bd74429cf.png) implies that
implies that 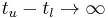 , however
, however 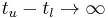 does not imply infinite many events in it.
does not imply infinite many events in it.
A timed language over an event set 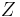 and a timed interval
and a timed interval ![[t_l, t_u]](/2012-wikipedia_en_all_nopic_01_2012/I/38d72adb0dfab9d43f3efa610f1b58fc.png) is a set of event segments over
is a set of event segments over 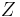 and
and ![[t_l,
t_u]](/2012-wikipedia_en_all_nopic_01_2012/I/38d72adb0dfab9d43f3efa610f1b58fc.png) . If
. If 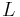 is a language over
is a language over 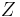 and
and ![[t_l, t_u]](/2012-wikipedia_en_all_nopic_01_2012/I/38d72adb0dfab9d43f3efa610f1b58fc.png) , then
, then ![L
\subseteq \Omega_{Z, [t_l, t_u]}](/2012-wikipedia_en_all_nopic_01_2012/I/deae54514aa1ddedd74076bcfb719d23.png) .
.
References
- [Zeigler76] Bernard Zeigler (1976). Theory of Modeling and Simulation (first ed.). Wiley Interscience, New York.
- [ZKP00] Bernard Zeigler, Tag Gon Kim, Herbert Praehofer (2000). Theory of Modeling and Simulation (second ed.). Academic Press, New York. ISBN 978-0127784557.